Forecasting with Bayesian VARs
Does Larger Mean Better?
Keywords:
Bayesian VARs, Macroeconomic Forecasting, Model SpecificationAbstract
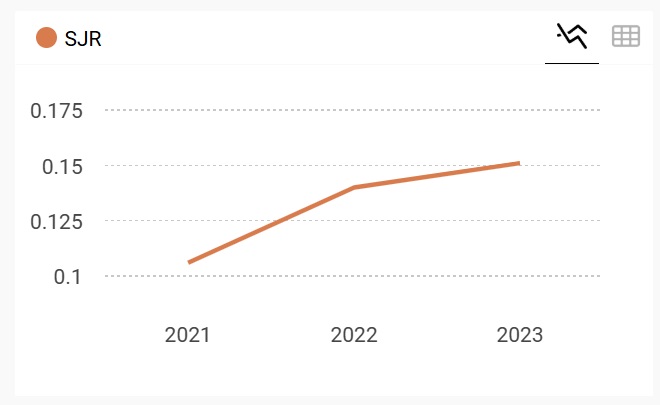
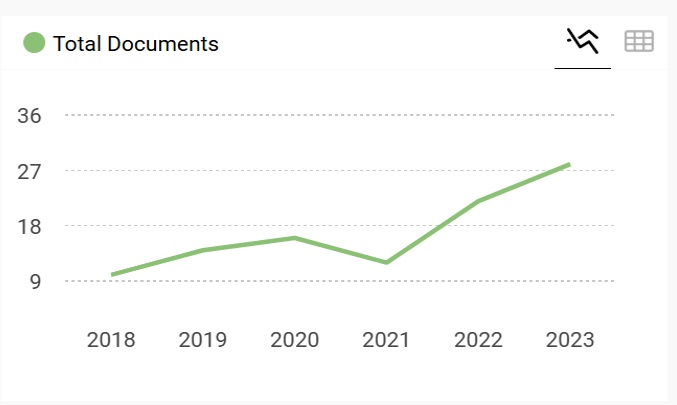
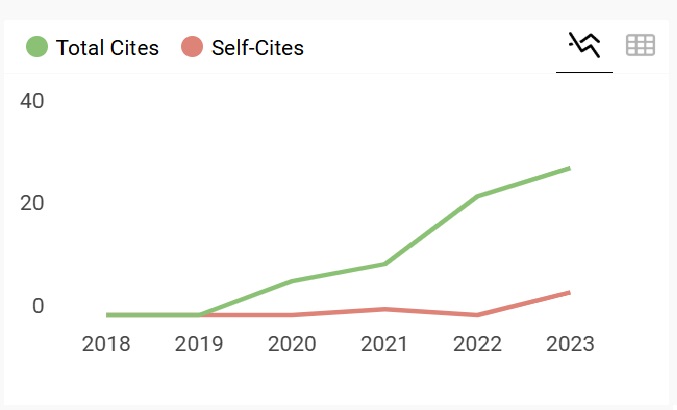
Conceptually, the impressive forecasting performance of the Bayesian VARs may be further improved by expanding the number of variables into the models. This paper compares the forecasting performance of a large 131 variable Bayesian VAR to much smaller models. Our paper gives especially careful consideration to the effect that a hyperparameter governing the overall tightness of the prior distribution can have, since the performance of a Bayesian regression can be so affected by it. Our results support the idea that larger Bayesian VARs perform better than smaller ones. However, when the hyperparameter of the prior distribution of a smaller model is carefully chosen, the improvement in performances of larger models is not as impressive as previously thought. Even a 3-variable model with an appropriately chosen shrinkage parameter will produce much better forecasts than those reported in the literature.
References
2. Bernanke, B.S., J. Boivin, and P.S. Eliasz (2005). Measuring the effects of monetary policy: A factor-augmented vector autoregressive (FAVAR) approach. The Quarterly Journal of Economics 120(1), 387 – 422.
3. Bernanke, B.S., and J. Boivin (2003). Monetary policy in a data-rich environment. Journal of Monetary Economics 50(3), 525 – 546.
4. Christiano, L.J., M. Eichenbaum, and C.L. Evans (1999). MNonetary policy shocks: What have we learned and to what end? In J.B. Taylor and M. Woodford (Eds.), Handbook of Macroeconomics, Volume 1, Chapter 2, pp. 65 – 148. Elsevier.
5. D’Agostino, A. and D. Giannone (2007). Comparing lternative predictors based on large-panel factor models. CEPR Discussion Papers 6564, Center of Economic Policy Research.
6. Forni, M., M. Hallin, M. Lippi, and L. Reichlin (2000). The generalized dynamic-factor model:Identification and estimation. The Review of Economics and Statistics 82(4), 540 – 554.
7. Forni, M., M. Hallin, M. Lippi, and L. Reichlin (2003). Do financial variables help forecasting inflation and real activity in the Euro area? Journal of Monetary Economics 50(6), 1243-1255.
8. Kadiyala, K.R. and S. Karlsson (1997). Numerical methods for estimation and inference in Bayesian VAR-models. Journal of Applied Econometrics 12(2), 99 – 132.
9. Litterman, R.B. (1986). Forecasting with Bayesian vector autoregressions – Five years of experience. Journal of Business and Economic Statistics 4(1), 25 – 38.
10. Magnus, J.R. and H. Neudecker (1999). Matrix Differential Calculus with Applications in Statistics and Econometrics (2nd ed.). John Wiley & Sons.
11. Robertson, J.C. and E.W. Tallman (1999). Vector autoregressions: Forecasting and reality.
12. Federal Reserve Bank of Atlanta Economic Review 84(1), 4 – 18.
13. Stock, J.H. and M.W. Watson (2002a). Forecasting using principal components from a large number of predictors. Journal of American Statistical Association 97, 1167 – 1179.
14. Stock, J.H. and M.W. Watson (2002b). Macroeconomic forecasting using diffusion indexes. Journal of Business and Economic Statistics 20(2), 147 – 162.
15. Stock, J.H. and M.W. Watson (2005). Implications of dynamic factor models for VAR analysis. NBER Working Papers 11467, National Bureau of Economic Research.
16. Zha, T. (1998). A dynamic multivariate model for use in formulating policy. Federal Reserve Bank of Atlanta Economic Review 83(1), 16 – 29.